分数加法 — 不同分母的情形
What are the results of the following two fraction additions?
a) (1 ⁄ 2) + (1 ⁄ 3) = ?
b) (1 ⁄ 2) + (2 ⁄ 3) = ?
The solution is right here!
a) (1 ⁄ 2) + (1 ⁄ 3) = (3 ⁄ 6) + (2 ⁄ 6) = (5 ⁄ 6)
b) (1 ⁄ 2) + (2 ⁄ 3) = (3 ⁄ 6) + (4 ⁄ 6) = (7 ⁄ 6)
All one needs is the concepts and operation of fractions. To elaborate, you need to know how to find the least common multiples.
Once the question a) was given to a student, and he got a surprising result (the teacher was surprised):
a’) (1 ⁄ 2) + (1 ⁄ 3) = 2 ⁄ 5
He (the student) has the following argument: (1 ⁄ 2) is to take one piece from two pieces, and (1 ⁄ 3) is to take one piece from three pieces, so in total there are 5 pieces and out of them we take 3. That’s 3 ⁄ 5!
This is obviously not the rule we are used in doing the arithmetic. And the teacher was persuading the student the result is not correct by turning all fractions into decimals:
1 ⁄ 2 = 0.5, 1 ⁄ 3 = 0.333…., and 2 ⁄ 5 = 0.4,
Note 0.5 + 0.333…. ≠ 0.4, therefore
(1 ⁄ 2) + (1 ⁄ 3) ≠ 2 ⁄ 5.
But this does not explain why the student’s argument is incorrect. If you were the teacher, how would you have explained the reason?
If you can do this, you can explain (b) similarly.
学生在a’) 中的计算是不对的。但是他给出了他的理由。你能告诉他为什么按算术规则不这样算吗?
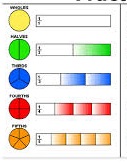
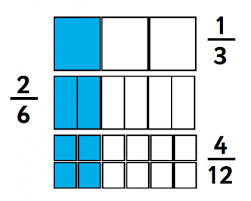
For your convenience, we show you the reference diagram for 1 ⁄ 2 and 1 ⁄ 3, as below.
上面是关于分数的几个直观表示图。你可以使用他们来做解释。